What AP Calculus Students Should Know for their Unit 4 Review

Need extra practice for your AP Calculus Unit 4 review? Outlined are the topics and derivative application practice problems aligned with College Board’s curriculum to study for a Unit 4 test.

This unit brings to light the reason we learned the derivative rules from Unit 2 and Unit 3. It showcases real-life applications of motion and other rates of change.

As College Board outlines, the topics to review for Unit 4 are:

· Interpreting the Meaning of the Derivative in Context

The key in Unit 4 is that “rate of change” implies derivative. The derivative is the instantaneous rate of change in several contexts like air filling a balloon, velocity of driving a car, or coffee dripping into a pot.

Learning the units of measure in context is important in this section. To make that easier, remember that you’re looking for the change in y (or f or g, representing the dependent variable) over the change in x (or t, representing the independent variable). There’s no need to simplify the units so if the unit for y is meters/sec and the unit for x is seconds, the derivative would be in meters/sec/sec, or if you chose to simplify, meters/sec^2.

Try the following practice problem. Worked out solutions to these problems will be at the end of the post.

1. The temperature of a lake is modeled by T(m), where T is measured in degrees and m is months after January. Interpret T’(8)= -2.

· Straight-Line Motion: Connecting Position, Velocity, and Acceleration

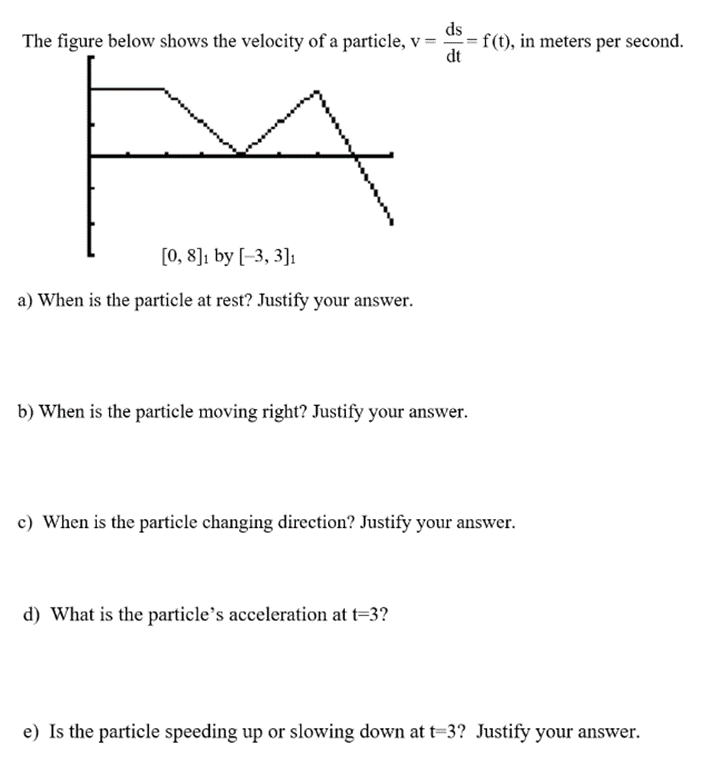
The derivative of position is velocity. The derivative of velocity is acceleration. “Velocity” implies instantaneous velocity (derivative) where average velocity would be the average rate of change of position.

Other important particle motion ideas: a particle is at rest when v(t)=0. A particle changes direction when v(t) changes signs. A particle is speeding up if the velocity and acceleration are the same sign at that time; a particle is slowing down if the velocity and acceleration are opposite signs at that time (some teachers wait until Unit 5 to discuss speeding up and slowing down).

Because these questions can be difficult given a position function, a velocity function, a graph, or a table, I start by writing the justification for each part THEN go into the context of the graph.

2.

· Rates of Change in Applied Contexts other than Motion

Every year on the AP Calculus exam, there is a free response question about a rate of change in context. Sometimes they are represented in functions, other times they are represented in tables. Try the AP Calculus AB/BC #1 from 2021 below. This is only part (a) as other parts of the question require knowledge not yet covered.

3.

· Introduction to Related Rates

This section and the next section could be combined but the difference is that this section focuses on applying the mathematical rules and procedures. The next section is interpreting and explaining the meaning of the solutions. I chose two full problems below, both setting up and solving the related rates problems. If you want practice with just this introduction section, check out Flipped Math.

I recommend starting these problems by identifying key information: state the variables that are changing (converting from verbal to mathematical forms). Next, determine a relationship (equation) between the variables. Then, take the derivative of that equation. Be aware of chain rule, product rule, and quotient rule when taking the derivative, along with implicit differentiation since every variable is changing with respect to time!

· Solving Related Rates Problems

From released AP Calculus exams, try the following problems.

4.

5.

· Approximating Values of a Function Using Local Linearity and Linearization

If you zoom in on a differentiable function, it eventually becomes a straight line hence “local linearity.” That’s why we can use tangent lines to approximate values near the point of tangency. Tangent lines can be used to approximate values like √80. We know that √81=9 so we can create a tangent line to f(x)= √x at x=81. Plugging 80 into our created tangent line will provide an estimate, and we can even take it a step further and determine if this is an over-estimate or under-estimate by looking at the concavity of f(x) at x=81. The concavity of f(x) is f’’(x) so you can find the second derivative and evaluate f’’(x) at x=81. Since f’’(81)<0, our estimate of √80 would be too large, an over-estimate.

6. Use a tangent line at x=1 to find the approximation of y=ln(x) at x=1.2. Is this an over- or under-estimate? Justify your answer.

· Using L’Hospital’s Rule for Determining Limits in Indeterminate Form

The indeterminate forms assessed on the AP exam are 0/0 and ∞/∞ (BC may cover more indeterminate forms similar to a Calculus 2 college course, but for the AP exam, you only need to know these two).

On the AP exam, it is crucial to show the rule applies in order to earn all points on the free response portion. While it’s important to understand that 0/0 and ∞/∞ indicate an indeterminate form, since it doesn’t represent equality in the value of the limit, it is incorrect to write that the limit = 0/0, for example. The student should separately show the limit of the numerator goes to 0 and the limit of the denominator goes to 0, then apply L’Hospital’s rule. This is illustrated in the solution to the problem below.

7.

AP Calculus Unit 4 Review

For your AP Calculus Unit 4 review, make sure you are practicing derivative problems from multiple perspectives: given functions, tables, graphs, and word problems. If you need extra practice, check out the 8 best resources to study for AP Calculus tests. These resources have questions that match the rigor of your tests.

If you need further explanation on how to approach some of these difficult Unit 4 review questions, especially when all of the topics are mixed together, consider individual Calculus tutoring with me. I answer any questions students have, then provide practice solving my past test questions and previous AP exam questions. Getting more practice with problems given graphs, tables, and word problems will help you be prepared for in class tests and the AP exam.

Sign up for individual tutoring today!

Solutions to the above problems:

1. The temperature of a lake is decreasing 2 degrees/month when t=8 (in September).

Note: t=0 would be January, t=1 would be February, and so on. Also notice, I omitted the negative sign since "decreasing" implies a negative rate. Alternatively, you could say "The temperature of the lake is changing -2 degrees/month in September."

2.

3.

4.

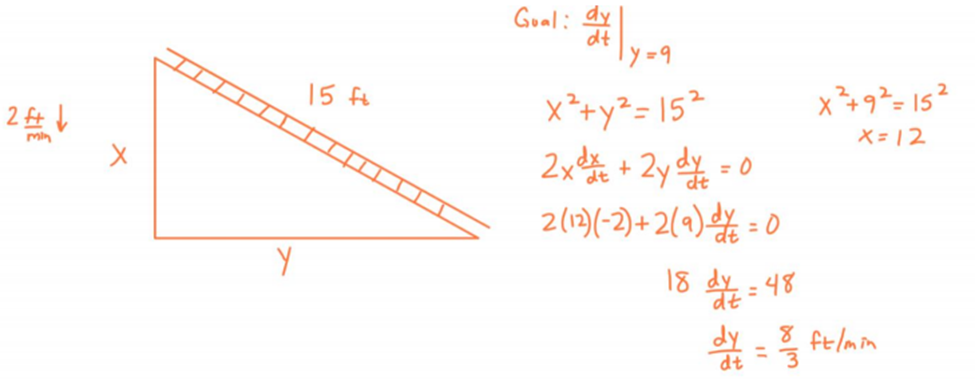
5.

Remember dr/dt = r’(t), so that -5 comes from the provided table, r’(3)=-5.

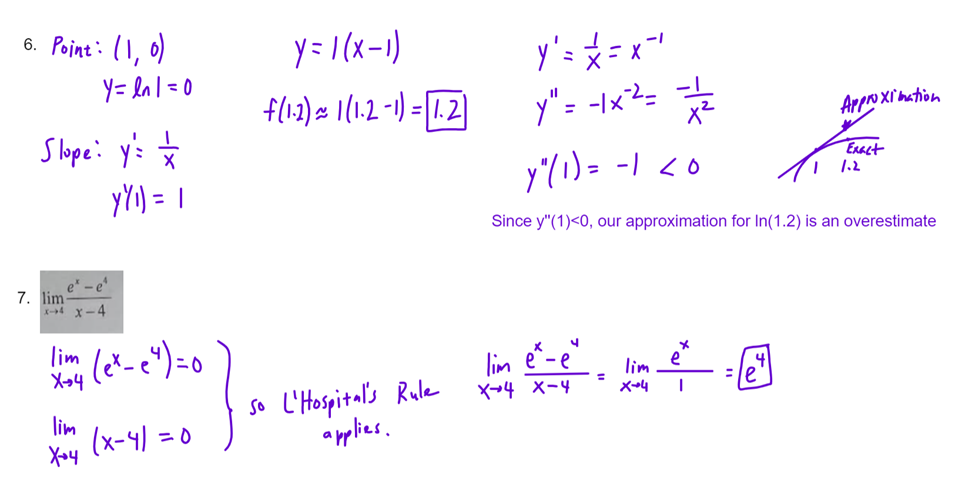
It’s worth noting that this particular problem doesn’t necessarily require L’Hospital’s Rule. You may recognize this is the alternate definition of derivative and you can evaluate the limit that way. The AP exam will write problems in a way that do require L’Hospital’s Rule, though.

AP® is a trademark registered by the College Board, which is not affiliated with, and does not endorse, this website.